[/caption]
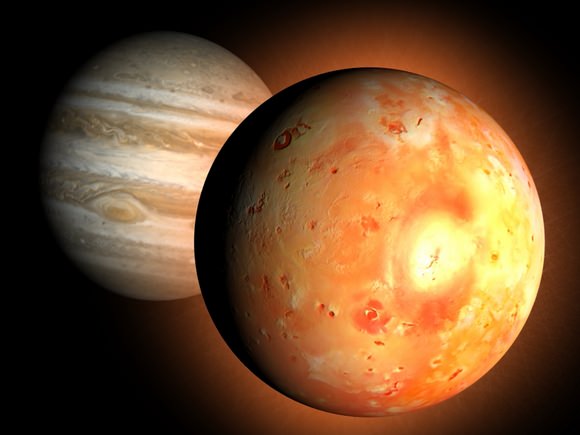
Io may be close to thermal equilibrium, according to a study published this week in Nature. And if the new findings are correct, the volcanically wild moon could one day break free of Jupiter’s hold — and lose its rare, volcanic splendor.
Io is Jupiter’s innermost moon, and is the most volcanically active body in the Solar System. Its geological activity is thought to be the result of tidal heating from friction generated by the pull of Jupiter’s gravity. But it’s not known whether this internally generated tidal heat is high enough to generate the heat flow observed on Io’s surface.
Using astronomic observations made between 1891 and 2007, Valery Lainey, of the Observatoire de Paris in France, and colleagues have estimated the dissipation of tidal energy in Io by tracking its effect on the orbital motions of the innermost Galilean moons. For Io, the value is in good agreement with the observed surface heat flow and suggests that Io is close to thermal equilibrium. Jupiter’s tidal dissipation is close to the upper bound of its average value, as would be expected from the long-term evolution of the system.
“The measured secular accelerations indicate that Io is evolving inwards, towards Jupiter,” Lainey and her colleagues add, “and that the three innermost Galilean moons (Io, Europa and Ganymede) are evolving out of the exact Laplace resonance.”
In an accompanying editorial, Gerald Schubert of the University of California in Los Angeles, writes that “Io’s orbital imprisonment is the cause of its spectacular volcanism.”
“If it eventually breaks free, the most volcanically active object in our Solar System will become dormant.”
Source: Nature

: Metis? Adrastea? Amalthea? Thebe?¹
Io may be the innermost Galilean satellite, but not the innermost Jovian moon. Amalthea’s discovery even predates the 20th Century.
¹ Moons of Jupiter found at Wikipedia.
Hm… How would Io break loose from Jupiter just like that? Jupiter’s Hill sphere is HUGE, and Io is deep within it; I can’t imagin it breaking loose without basically destroying the whole jovian satellite system. And besides, Io is quite massive, being about the same mass as Callisto and twice as heavy as Europa. Not an easy object to move.
Jorge, I’m not sure I understand your question. For one thing, Io is already moving relative to Jupiter and the other moons.
As regards disrupting orbits, apparently, from the article, Io is in orbital resonance, and already in the process of breaking free of that. If its continuing orbit around Jupiter but outside of resonance will disrupt other orbits I don’t know. (Note that apparently also Europa, with its tidal heated ocean, and Ganymede is also already in the process of changing orbits.)
I don’t quite understand either, but that wouldn’t be the first time.
The title of the article, and the first paragraph, states that Io could leave Jupiter’s hold.
The fourth and fifth paragraph says Io is moving inwards toward Jupiter (but out of its Laplace orbital resonance).
How can Io move inward and out of Jupiter’s gravity well at the same time? Wouldn’t an inward trek make tidal forces greater? What am I missing here?
(Also, there are two quotes in this article that are exactly the same. Perhaps there is a cut and paste editing error?)
Oh, now I see the confusion in the text. The wording is misleading, I agree. I suppose, if Io breaks free it will experience less heating. Jupiter’s tidal effect on Io can then be relaxed by Jupiter-Io interaction by its lonesome. Today the other mentioned moons hold Io back by the resonance, thus making the tidal stresses worse. [Wikipedia claims its a nice 1:2:4 resonance.)
And of course when the last two moons (I guess not Io) breaks free from their mutual resonance, _they_ will sort of affect each other more. But as I said, dunno what Io free on its lonesome will do.
Torbjorn, it’s one thing to move slightly closer or farther away relative to a satellite’s primary. That happens all the time, with tidal transferences of momentum between the primary and the satellites. Quite another thing is for a satellite to completely break free from such a strong gravity well as Jupiter’s. That would need some major (and I mean major) interactions between Io and all the other jovian satellites, completely disrupting the system, and probably sending one or more of Io’s siblings into Jupiter.
Maybe I’m wrong, but I can’t see this happening just like that.
It is certainly possible for this to happen in principle. AFter all I think that Earth and the other terrestrial planets are being nudged out by their interaction with Jupiter. Of course Jupiter as a result is nudged slightly inwards as a result. This process conserves everything, energy and angular momentum in particular.
The four Jovian satellites are a mini-solar system of sorts, except Jupiter is not a star. So it is certainly possible that 5-body interactions of Io, one with Jupiter and the rest with the other moons can certainly nudge Io outwards. Yet I wonder by how much. If Jupiter had a Neptune mass orbiting around it further out (a double gas giant — maybe possible out there) then this larger body has lots of energy and angular momenta to transfer to its light weight cousins further in.
There are of course resonance conditions. In the perturbation expansion there is a first order perturbative term which is a ratio of frequencies, that can go singular at exact resonant conditions. Interestingly the Earth and Jupiter have a 1:11.8 periodic relationship which is nearly resonant. This is common throughout the solar system. In about 1 billion year the ratio of Earth-Jovian orbital periods will be 1:11.9 — slightly closer to resonance. In another billion years resonance will be reached!
What happens if orbital drift reaches exact resonance? This is a sticky mathematical problem. It is one which Kolmogorov, Arnold and Moser addressed in the 1960’s in their celebrated KAM result. Near resonance the energy surface for dynamics becomes chaotic as the so called KAM surface is disrupted or “ounctured.” The KAM surface is the limit of irrational winding. At resonance the dynamics becomes completely chaotic. However, this chaos is brief and shoves the planets off resonance and some relative order is restored — though what that is is not really predictable. So except for some highly symmetric situations an orbtial system can pass through resonance with only a rather brief period of chaotic motion that is not likely to be disasterous.
There are some highly symmetric models with 3 or 4 bodies where one body can end up expelled from the gravitational system with infinite velocity. Remember this is the domain of pure classical mechanics so we are ignoring relativity.
So back to the case of Io, clearly something like this must happen. The physics is much more complicated for this many bodies and the techniques involve prolongation of Lie symmetries for the orbits. It is really serious mathy stuff — outside of my interests and beyond my patience for calculation..
The tag line on this article is misleading. All it says is that Io could ‘break free’. It doesn’t say it could break free of orbiting Jupiter.
Io’s tidal heating is due to its orbital eccentricity which is maintained by the Laplace resonance with Europa and Ganymede. All that is necessary for Io to ‘break free’ of its ‘orbital imprisonment’ and ‘go dormant’ is for that resonance to be disrupted.
Hm… yeah, that makes sense. I assumed that “break free from Jupiter’s hold” meant leaving jovian space alltogether.
This makes a little more sense. So the upshot is that Io is nudged out enough to halt the tidal heating of the moon so the extreme volcanism is halted.